There are some punters (and tipsters) who advocate a money management strategy, which involves the incremental increase of stakes after lost bets, in an attempt recover previously lost money.

It is frequently regarded by such proponents as a fail-safe strategy on the grounds that it is inevitable that one will win in the end, and when one does so, all previously lost money will be recovered together with the originally targeted profit from the first bet.

The more astute amongst you will have already spotted the flaw: nothing is inevitable in gambling. If there was, it wouldn’t be gambling. The reason some players ignore the flaw is on account of a couple of heuristic biases: overconfidence (that they will win) and underestimating the probabilities of losing streaks. This type of gambling money management is traditionally known as the Martingale system.

The Martingale Strategy

The Martingale staking plan comes from the world of casino gambling, and in particular the game of roulette. A popular game at the roulette wheel is red-black, where the gambler must decide whether the ball will land on either a red or a black number after each spin.

Overlooking the influence of the house edge, the odds of either result are 2.00. The idea behind the basic Martingale strategy is to double the stake size after each losing wager, and return to the starting (or baseline) stake after every win, although one can apply it to any betting odds using the expression:

Martingale rate of progression = odds / (odds - 1)

For example, if betting odds of 3.00, the rate of progression of stake increase would be 1.5.

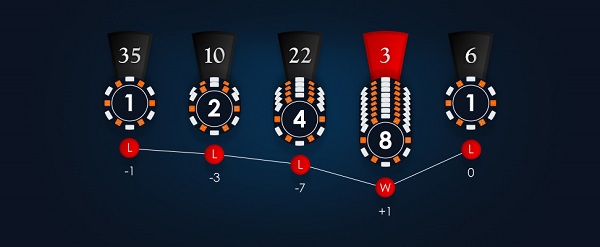
In this way, previous losses are recovered after each successful result plus the original targeted profit, as the following sequence of wheel spins reveals.

Wheel spin	Bet	Stake	Outcome	Result	Profit	Running total
1	Red	1	Black	Lose	-1	-1
2	Red	2	Black	Lose	-2	-3
3	Red	4	Black	Lose	-4	-7
4	Red	8	Red	Win	+8	+1
5	Red	1	Black	Lose	-1	0
6	Red	2	Red	Win	+2	+2
7	Red	1	Red	Win	+1	+3
8	Red	1	Black	Lose	-1	+2
9	Red	2	Black	Lose	-2	0
10	Red	4	Red	Win	+4	+4

Martingale changes risks, not mathematical expectations

In his e-book Successful Staking Strategies (2001), Stuart Holland provided a simple but excellent demonstration of why Martingale is unable to make something out of nothing.

Consider the first 3 wheel spins in the sequence above. The 3 consecutive losing blacks represent just 1 of 8 possible outcomes, each of which is as likely as any other.

The table below shows the profit expectation for each of these 8 permutations, where R=Red and B=Black, discounting the influence of the house edge (in the form of the green zero). To calculate the expectation for any outcome, one simply multiplies the actual profit or loss for that outcome by the probability of it occurring.

Permutation	Bet	Outcome	Stakes	Profit	Total	Chance	Expectation
1	R, R, R	B, B, B	1, 2, 4	-1, -2, -4	-7	0.125	-0.875
2	R, R, R	B, B, R	1, 2, 4	-1, -2, +4	+1	0.125	+0.125
3	R, R, R	B, R, B	1, 2, 1	-1, +2, -1	0	0.125	0
4	R, R, R	B, R, R	1, 2, 1	-1, +2, +1	+2	0.125	+0.25
5	R, R, R	R, B, B	1, 1, 2	+1, -1, -2	-2	0.125	-0.25
6	R, R, R	R, B, R	1, 1, 2	+1, -1, +2	+2	0.125	+0.25
7	R, R, R	R, R, B	1, 1, 1	+1, +1, -1	+1	0.125	+0.125
8	R, R, R	R, R, R	1, 1, 1	+1, +1, +1	+3	0.125	+0.375

Summing the individual expectations for the 8 permutations gives the total expectation for the strategy. It is zero. Hence, for a fair roulette wheel, all we can hope for over the long term is to break even.

Of course, real roulette wheels aren’t fair; a single game of black-red in a casino comes with negative expectation, and so, therefore, does the sum of lots of games.

A similar analysis for level staking (where all stakes are the same size) returns exactly the same result: an overall expectation of zero.

Permutation	Bet	Outcome	Stakes	Profit	Total	Chance	Expectation
1	R, R, R	B, B, B	1, 1, 1	-1, -1, -1	-3	0.125	-0.375
2	R, R, R	B, B, R	1, 1, 1	-1, -1, +1	-1	0.125	-0.125
3	R, R, R	B, R, B	1, 1, 1	-1, +1, -1	-1	0.125	-0.125
4	R, R, R	B, R, R	1, 1, 1	-1, +1, +1	+1	0.125	+0.125
5	R, R, R	R, B, B	1, 1, 1	+1, -1, -1	-1	0.125	-0.125
6	R, R, R	R, B, R	1, 1, 1	+1, -1, +1	+1	0.125	+0.125
7	R, R, R	R, R, B	1, 1, 1	+1, +1, -1	+1	0.125	+0.125
8	R, R, R	R, R, R	1, 1, 1	+1, +1, +1	+3	0.125	+0.375

Take a closer look at the two tables. The Martingale strategy has increased the number of times we can expect to make a profit, relative to a level staking strategy, from an individual play, in this example from 4 to 5.

Unfortunately, this is at the expense of one large loss. All Martingale has really achieved is a change in the distribution of risks. The trade off for gaining one extra outcome with positive expectation is another with a much greater negative expectation, relative to the equivalent outcome for level staking. This is the source of the inherent danger associated with the strategy.

Using Martingale

In sports betting, the Martingale might seem to offer the punter a chance of profiting even where he is unable to secure positive expected value, since each win will recover his preceding losses and add a little extra each time.

The preceding analysis, however, will hopefully have convinced you that the Martingale progression is both mathematically flawed and inherently very risky, since any extended run of consecutive losses will soon increase the stake size to very high levels. 10 even-money losses in succession, for example, will require the 11th stake to be 1,024 units, just to win 1.

Depending on the size of stake you began with, conceivably this might be beyond the accepted limits of the bookmaker. Equally, it might be larger than the size of your remaining bankroll.

Underestimating the chances of losing runs

Just how likely is it to have a streak of 10 consecutive losses at even money? In isolation, the mathematics to calculate this is easy. If each independent bet has a 50% (or 0.5) probability of loss, the probability of 10 sequential losses will be given by 0.510 = 0.0977%.

Such a low probability fools many into believing that Martingale is a relatively safe strategy to follow. But what is the probability of having such a losing streak at some point during a series of a much larger number of wagers?

The mathematics for this calculation is far trickier, but intuitively we can recognise that it will be far more probable than the quoted percentage for an individual streak, since there are many more opportunities for it to happen. Fortunately, there is a very useful way of estimating the longest losing streak we might expect to see during a long series of wagers.

S_L=(Ln(N))/(Ln(O_L))

S_L is the length of the expected maximum losing streak, N is the total number of bets placed, ‘Ln’ is the natural logarithm (available on any scientific calculator) and O_L is the odds of losing an individual bet, which can be calculated from the betting odds, or odds for winning, O_W, by:

O_L= O_W/(O_W- 1)

So for example, in a series of 1,000 bets at fair odds of 2.00, we would typically expect at least one streak of 10 consecutive losses. As we’ve seen, such a streak will mean that the next stake will need to be 1,024 times bigger than the first one.

To reasonably be able to cope with such losing streak expectation, the proportional sizes of your bankroll and baseline stake will need to be appropriately calculated. The longer your betting series is, the smaller the baseline stake as a proportion of your bankroll will need to be to cope with worst case scenarios.

For a series of 1,000 even-money wagers, your bankroll should arguably be at least 1,000 times bigger than your baseline stake size. Either this will mean baseline stakes (and consequently profits after wins) of such small magnitude that it’s hardly worth the bother of following the strategy, or running the risk of losing considerably large sums of money.

Risk of bankruptcy

In my book Fixed Odds Sports Betting: Statistical Forecasting and Risk Management (2003), I tested the Martingale strategy for a real-world betting series of 250 wagers with an average individual win expectancy of 0.5 (i.e. odds of 2.00).

For baseline stakes of 1% of the initial bankroll, the probability that one would end up bankrupt assuming that the odds were fair was 53%. For an equivalent level staking strategy, the percentage was so small as to be effectively 0%. For a scenario where the bookmaker held a 5 and 10% advantage respectively over the punter, the bankruptcy risk for Martingale rose to 65% and 78%.

Even for scenarios where the punter held the advantage, there was still a considerable risk. With a 5% advantage it was still as high as 38%. Of course, where punters have secured positive expected value through their skill of forecasting, one might wonder why they would need to chase their losses in the first place.

An illusion

Theoretically, with infinite wealth, infinite number of bets, infinite time and an infinitely accommodating bookmaker, it could be argued that the Martingale becomes a winning strategy.

Except, of course, you cannot increase infinite wealth, and we might reasonably question the motivation for trying if one already possessed it. In the real world of gambling and betting, the bottom line with Martingale is this: if you’re not good enough to beat the odds, Martingale offers the surest possible way to financial ruin; and if you are, you don’t need it anyway.

The apparent ability of Martingale to turn losses into profits is, quite simply, an illusion, and a very risky one at that.

MORE: TOP 100 Online Bookmakers >>>

MORE: TOP 20 Bookmakers that accept U.S. players >>>

MORE: TOP 20 Bookmakers that accept Cryptocurrency >>>

Source: pinnacle.com