Betting tipsters often promise high strike rates that will win you a fortune. Although the promise of big profits is alluring, how can you tell the difference between a profitable tipster and a salesman. Read on to find out the two factors you need to evaluate before you decide which expert picks to trust.
In a previous article, Mark Taylor explained how luck influences betting and whether profit is always a sign of skilful betting. Using a series of coin tosses as an example, Mark used a binomial calculator to calculate the probability of making a profit after ten consecutive wagers.
The binomial distribution is well suited to 50-50 propositions like point spread or Asian handicap markets, where the odds for each side are close to even money, or a little shorter after the bookmaker applies his betting margin. Often, however, bettors bet on all sorts of different prices with all sorts of different stakes, for example 1X2 markets in soccer or match betting in tennis.
In such circumstances, we can rely on what is known as the t-distribution and the student’s t-test for statistical significance, which uses it. In this article, I explain how to use the t-distribution to gauge the performance of a betting tipster.
The length of a tipster’s record
The t-distribution is very similar to the bell-shaped normal distribution and for numbers of wagers above about 30, is to all intents and purposes the same thing. The t-test investigates the likelihood that a profit from a series of wagers could have happened by chance.
A return of 120% from 100 wagers at odds of 10.00 or longer is most likely to be a consequence of luck. Same returns from betting odds-on prices is an indication of skill.
The smaller the likelihood, the more probable it is that something else, like the bettor’s skill, is explaining their profitability. The t-test simply compares the bettor’s observed return to a theoretical expectation (assuming chance only) defined by the market they’re betting in.
Usually this would be a loss equivalent to the bookmaker’s margin, or break even if the bettor is taking the trouble to find best prices using an odds comparison tool. The resulting t-score is then analysed to determine whether the difference is statistically significant.
It should be intuitively obvious that the larger the profitability the bigger the t-score and more statistically significant the betting history, in other words, the more likely it is that skill has played a part. The t-score is directly proportional to a bettor’s excess average return over expectation.
Similarly, the longer the history (for an equivalent return) the more likely it is that something other than chance is at work. Consider, for example, two bettors with a 120% return on investment. The first achieved it from 10 wagers, the second from a 1,000. Who is more likely to be the skilled bettor?
If in doubt, think of tossing coins again. Landing six plus heads out of 10 tosses is obviously far more likely than landing 600 plus from 1,000 assuming only chance. Were one to land 600 or more heads, one might reasonably suspect the coin to be biased.
Similarly, we are more likely to conclude that a bettor with a long record of profitability is demonstrating skill. The t-score is in fact proportional to the square root of the number of wagers.
Long vs. short odds
Less intuitive is the influence of the betting odds. In fact, a 120% return on investment from betting odds around 1.25 will be a much better indicator of skill than an equivalent profitability from betting odds around 5.00. Betting on lower probability outcomes (longer odds) is inherently riskier (assuming equivalent stakes) because it is more at the mercy of random variability.
To put it another way, returns are more volatile. 19 or 21 winners at odds of 5.00 will give returns of 95% or 105% respectively. In contrast, 79 or 81 winners at odds of 1.25 will show 98.75% or 101.25% profit over turnover. Betting longer odds implies taking more risk to get more reward.
We can see the influence of the betting odds by means of the standard deviation in profits and losses of the betting history. For level staking, the standard deviation can be approximated by the following expression.
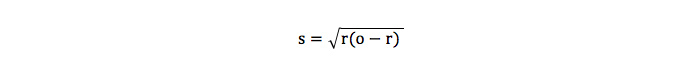
Where o represents the average odds for the betting history and r is the bettor’s actual return. The standard deviation in profits and losses betting at odds of 5.00 is more than eight times larger than when betting at odds of 1.25. Assuming that expected returns (based on luck only) are 100% (or break even), the t-score is given by the following equation:
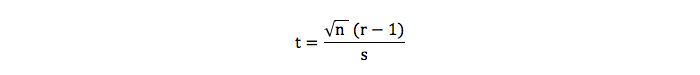
Where n is the number of wagers. Consequently, the t-score for equivalent returns and length of betting history will be more than eight times smaller betting at odds of 5.00 compared to 1.25.
It should be clear that superior yields achieved through betting longer odds, as is typical in markets like horse racing, are not necessarily a sign of better forecasting talent. The same amount of luck will deliver much bigger percentage returns.
Hence, comparisons of betting histories that consider only percentage returns, as is common when ranking tipsters, are fundamentally misleading. By taking into consideration the betting odds, the t-score provides a measure of the quality of the risk-adjusted return in excess of expectation.
Calculating chance
The final step is to convert the t-score into a probability (the p-value) that a history of profitability could arise by chance only. Those with Microsoft Excel can make use of the TDIST function. This takes the form TDIST (t, degrees of freedom, tails), where t is the t-score whilst degrees of freedom is the number of independent pieces of data and is equivalent to the number of wagers minus one.
The tails argument can be either one (for the one-tailed t-test) or two (for the 2-tailed t-test). Since we’re really only interested in whether a profit is statistically significant, the former is preferred in this case. Alternatively, one can make use of an online calculator where these values can be inputted.
The table below shows some examples of t-scores and their corresponding p-values for betting histories of 100 wagers and a return on investment of 120%.
| Odds | t-score | p-value |
| 1.5 | 3.33 | 0.06% |
| 1.75 | 2.46 | 0.78% |
| 2 | 2.04 | 2.19% |
| 2.5 | 1.60 | 5.63% |
| 3 | 1.36 | 8.83% |
| 4 | 1.09 | 13.89% |
| 5 | 0.94 | 17.56% |
| 10 | 0.62 | 26.98% |
| 25 | 0.37 | 35.45% |
| 50 | 0.26 | 39.72% |
Evidently, the average odds at which someone bets has a big influence on whether their profitability can be considered lucky or skilful. A return of 120% from 100 wagers at odd of 10.00 or longer should clearly be considered largely a consequence of luck.
By contrast, if a bettor was to show the same return betting odds-on prices, it is far more likely that the profitability has arisen because of their skill. Consequently, when comparing betting histories, for example from tipsters, it is not enough just to analyse their percentage returns, but also the length of their records and the odds at which they were achieved.
MORE: TOP 100 Online Bookmakers >>>
MORE: TOP 20 Bookmakers that accept U.S. players >>>
MORE: TOP 20 Bookmakers that accept Cryptocurrency >>>
Source: pinnacle.com
